- Introduction: Understanding the Equation of Distinct Positive Integers
- The Search for Solutions: Strategies and Techniques
- Challenges and Limitations: Exploring the Complexity of the Problem
- Applications and Implications: Real-World Scenarios and Future Directions
Introduction: Understanding the Equation of Distinct Positive Integers
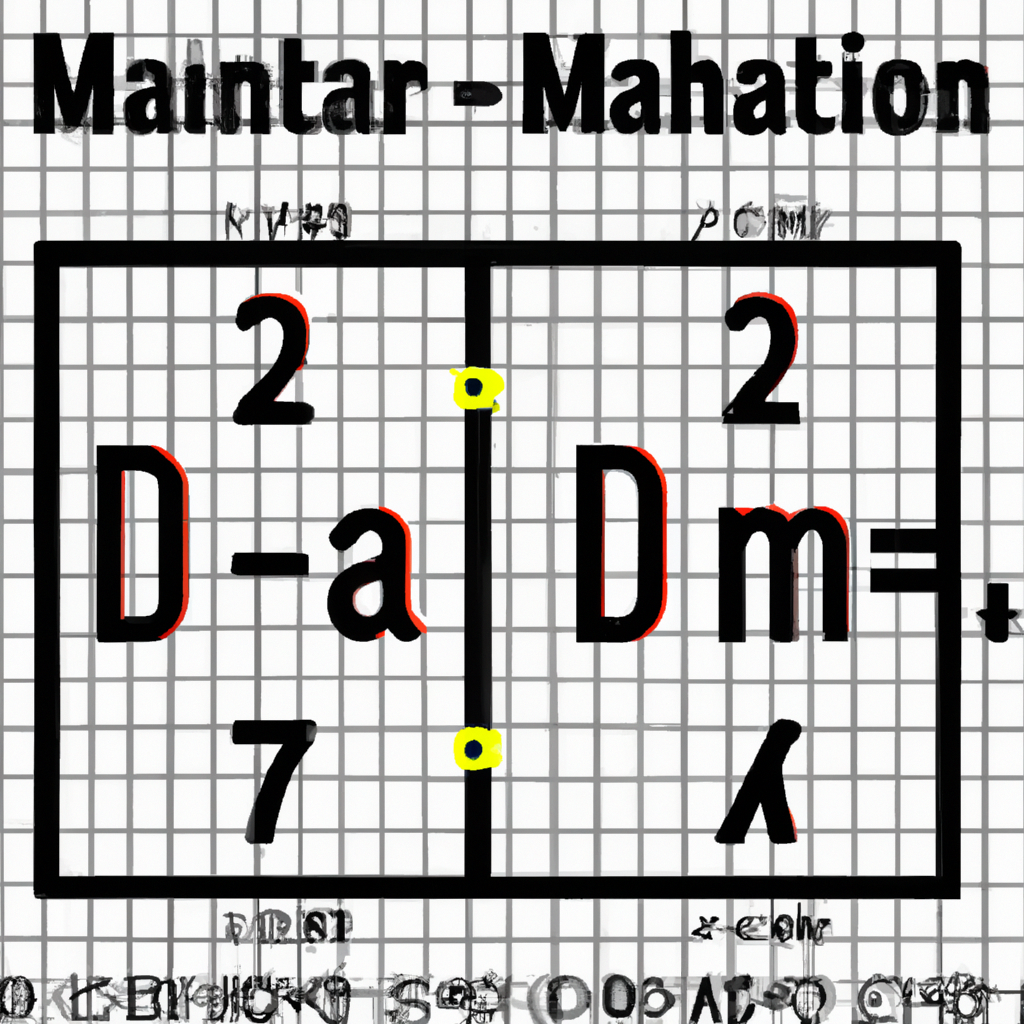
The equation of distinct positive integers is a mathematical problem that has puzzled mathematicians for centuries. It involves finding two positive integers that add up to a given number, but are not equal to each other. For example, if the given number is 10, the solution would be 3 and 7, since they are both positive integers that add up to 10, but are not equal to each other.
This problem may seem simple at first glance, but it becomes increasingly difficult as the given number gets larger. Mathematicians have been trying to find a general formula or algorithm that can solve this problem for any given number, but so far, no such solution has been found. Instead, mathematicians have developed various strategies and techniques to solve this problem for specific cases.
In this article, we will explore the equation of distinct positive integers in depth, looking at its history, its significance, and its challenges. We will also examine some of the strategies and techniques that have been developed to solve this problem, and discuss their limitations and implications. Finally, we will look at some real-world scenarios where this problem arises, and consider future directions for research in this area.
The Search for Solutions: Strategies and Techniques
One of the most common strategies for solving the equation of distinct positive integers is brute force. This involves trying every possible combination of positive integers that add up to the given number until a solution is found. While this method is simple and straightforward, it becomes impractical for larger numbers, as the number of possible combinations grows exponentially.
Another strategy is to use algebraic equations to represent the problem. For example, if the given number is n, we can represent the problem as x + y = n, where x and y are the two distinct positive integers we are trying to find. By manipulating this equation, we can derive other equations that help us narrow down the possible values of x and y. However, this method also has its limitations, as it can be difficult to find a general formula that works for all cases.
A more advanced technique is to use number theory to solve the problem. Number theory is a branch of mathematics that deals with the properties and relationships of numbers. By applying number theory concepts such as modular arithmetic, prime factorization, and Diophantine equations, mathematicians have been able to develop more efficient algorithms for solving the equation of distinct positive integers. However, these algorithms can still be computationally intensive for large numbers, and may not always provide a solution.
Challenges and Limitations: Exploring the Complexity of the Problem
The equation of distinct positive integers is a deceptively simple problem that hides a great deal of complexity. One of the main challenges is the sheer number of possible combinations of positive integers that need to be checked. As the given number gets larger, the number of possible combinations grows exponentially, making it impractical to use brute force methods.
Another challenge is the lack of a general formula or algorithm that can solve this problem for any given number. While mathematicians have developed various strategies and techniques to solve this problem for specific cases, there is no known formula that works for all cases. This means that each case must be approached individually, which can be time-consuming and labor-intensive.
Finally, there are limitations to the solutions that can be found. For example, there may be multiple solutions to the equation of distinct positive integers for a given number, but some of these solutions may not be useful or relevant in a particular context. Additionally, some solutions may be too large or too small to be practical, depending on the application. These limitations must be taken into account when solving the equation of distinct positive integers in real-world scenarios.
Applications and Implications: Real-World Scenarios and Future Directions
While the equation of distinct positive integers may seem like an abstract mathematical problem, it has many real-world applications. For example, it can be used in cryptography to generate secure keys for encryption and decryption. It can also be used in computer science to optimize algorithms and data structures. Additionally, it has applications in physics, chemistry, and engineering, where it can be used to model and solve various problems.
As for future directions, mathematicians are still actively researching the equation of distinct positive integers, looking for new strategies and techniques to solve this problem more efficiently. Some researchers are also exploring the connections between this problem and other areas of mathematics, such as number theory, combinatorics, and algebraic geometry. By deepening our understanding of this problem, we can unlock new insights and applications in a wide range of fields.
In conclusion, the equation of distinct positive integers is a fascinating and challenging problem that has captured the attention of mathematicians for centuries. While there is still much to be learned about this problem, the strategies and techniques that have been developed so far have already had a significant impact on various fields. As we continue to explore this problem and its applications, we can expect to see even more exciting developments in the future.
Andrey Bulezyuk is a Lead AI Engineer and Author of best-selling books such as „Algorithmic Trading“, „Django 3 for Beginners“, „#TwitterFiles“. Andrey Bulezyuk is giving speeches on, he is coaching Dev-Teams across Europe on topics like Frontend, Backend, Cloud and AI Development.
0 Comments